Gelöste Aufgaben/UEBI
Aufgabenstellung
Der Euler-Bernoulli-Balken AB wird durch seine Gewichtskraft belastet. Er ist in A fest eingespannt und hat eine konstante Breite b sowie eine zwischen A und B linear veränderliche Höhe h.
In UEBF haben wir eine Näherungslösung für dieses Problem berechnet.

Gesucht ist die analytische Lösung des Problems.
Gegeben sind für den Balken:
- Länge ℓ, Breite b,
- E-Modul E, Dichte ρ und
- die Höhe h0=b und h1 jeweils in A und B; dazwischen ist die Höhe linear veränderlich.
Lösung mit Maxima
Um zur analytischen Lösung zukommen, müssen wir berücksichtigen, dass
- .
Wir müssen also hier die Abhängigkeit der Querschnittseigenschaften von "x" in der Differentialbeziehung berücksichtigen. Das macht die Sache deutlich komplizierter als vorher.
Header
Wir haben die Differential-Beziehungen
für die Querkraft Q, das Moment M, die Verkippung der Querschnitte ϕ und die Auslenkung w. Dabei ist die ortsabhängige Streckenlast
Die Höhe des Balkens ist linear veränderlich, nämlich
- .
/*******************************************************/
/* MAXIMA script */
/* version: wxMaxima 18.10.1 */
/* author: Andreas Baumgart */
/* last updated: 2019-09-30 */
/* ref: TM-C, Balken mit linear-veränderlicher Höhe */
/* description: finds the analytic solution for */
/* problem */
/*******************************************************/
/* declare variational variables - see 6.3 Identifiers */
declare( "ℓ", alphabetic);
declare( "ϕ", alphabetic);
Declarations
Diese Abkürzungen führen wir ein:
- ,
- .
Für die Ergebnisse setzten wir dann exemplarisch
an - sonst werden die Ausdrücke zu umfangreich.
/* make equations of motion dim'less with load case #6 */
reference : [Phi[ref] = W[ref]/ℓ, W[ref] = q[ref]*ℓ^4/(8*E I[ref]),
M[ref] = m*g*ℓ, Q[ref] = m*g,
q[ref] = m*g/ℓ, EI[ref]=E*b*((H[0]+H[1])/2)^3/12];
/* system parameters */
params: [q[0] = A(xi)*rho*g,
A(xi) = b*h(xi),
I(xi) = b*h(xi)^3/12,
h(xi) = H[0]*(1-xi)+ H[1]*xi];
params: append(params,
solve((H[0]+H[1])/2*b*ℓ*rho=m, rho));
geometry : [alpha=1/2];
dimless: [x = xi*ℓ, H[0]=b, H[1]=alpha*b];
sections: [%c4=C[0], %c3=C[1], %c2=C[2], %c1=C[3]];
Dimensionless Form of Differential Equations
Beim Aufintegrieren der Differentialgleichungen stören die vielen dimensionsbehafteten Parameter. Viel einfacher werden die Gleichungen, wenn wir sie in dimensionsloser Form - mit dimensionsloser Auslenkung, Kippwinkel, Biegemoment und Querkraft anschreiben, also
- .
Wir wählen dazu als Referenzlösung den Kragbalken mit konstantem Querschnitt unter konstanter Streckenlast, mit der maximalen Auslenkung
- .
Als Referenz-Werte für die Streckenlast wählen wir hier die Werte unseres Balkens in x=ℓ/2, demnach
- .
Die Differentialgleichungen werden dadurch und mit der dimensionslosen Ortskoordinate
viel einfacher, nämlich
- .
Damit es übersichtlicher wird, lassen wir die Tilden über den gesuchten dimensionslosen Funktionen gleich wieder weg.
/******************************************************/
/* Boundary Value Problem Formulation */
/* field */
dgl : [ Q[ref]*diff(Q(xi),xi)/ℓ = - q(xi),
M[ref]*diff(M(xi),xi)/ℓ = + Q[ref]*Q(xi),
E*I(xi)*diff(Phi[ref]*ϕ(xi),xi)/ℓ = - M[ref]*M(xi),
diff(W[ref]*w(xi),xi)/ℓ = + Phi[ref]*ϕ(xi)];
dgl: subst(reference,dgl);
Integration Of Differential Equation
Die Differentialbeziehungen lösen wir nun sukzessive zu
- ,
- .
Bis hier ist alles wie gehabt - aber jetzt steht das ortsveränderliche Flächenmoment I(ξ) im Nenner. Maxima liefert
und im nächsten Schritt schließlich
- .
Darin enthalten sind die unbekannten - also gesuchten - Integrationskonstanten
- .
/******************************************************/
/* integrate differential equations */
displ : ratsimp(integrate(subst(dimless,ratsimp(subst(params,solve(dgl[1],Q(xi))))),xi));
displ : append(displ, ratsimp(integrate(subst(displ,solve(dgl[2],M(xi))),xi)));
displ : append(displ, ratsimp(
integrate(
ratsimp(subst(dimless,subst(geometry,subst(displ, subst(params,solve(dgl[3],'diff(ϕ(xi),xi))))))),xi
)));
displ : append(displ, ratsimp(
integrate(
subst(displ,
solve(dgl[4],w(xi))
),
xi)));
displ : ratsimp(subst(sections, subst(geometry,displ)));
Boundary Conditions
Diese Unbekannten bestimmen wir aus den Randbedingungen, nämlich
und damit
- .
/******************************************************/
/* part II: boundary conditions */
node[A]: [ w(0) = 0,
ϕ(0) = 0];
node[B]: [ Q(1) = 0,
M(1) = 0];
BCs : [subst(node[B],subst([xi=1],displ[1])),
subst(node[B],subst([xi=1],displ[2])),
subst(node[A],subst([xi=0],displ[3])),
subst(node[A],subst([xi=0],displ[4]))];
scale: [3, 9, 8, 4];
BCs : expand(ratsimp(scale*BCs));
Solving
Zum Lösen bringen wir die Gleichungen in die Form
- ,
die wir lösen zu
- .
/* integration constants = unknowns */
X : [C[0],C[1],C[2],C[3]];
ACM: augcoefmatrix(BCs,X);
/* system matrix and rhs */
AA : submatrix(ACM,5);
bb : - col(ACM,5);
/* print OLE */
print(subst(params,AA),"*",transpose(X),"=",subst(params,bb))$
/******************************************************/
/* solving */
D : ratsimp(determinant(AA))$
[ P, L, U] : ratsimp(get_lu_factors(lu_factor(AA)))$
cc : ratsimp(linsolve_by_lu(AA,bb)[1])$
sol : makelist(X[i] = cc[i][1],i,1,4)$
Post-Processing
Die Ergebnisse schauen wir uns in dimensionsloser Form an, wobei wir die Standard-Lösungen für den Balken unter konstanter Streckenlast ansetzen.
Für
finden wir
- ... für w(ξ):
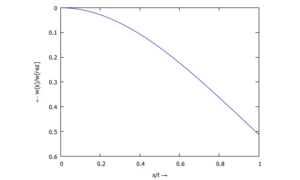
Auslenkung w(x) - ... für ϕ(ξ):
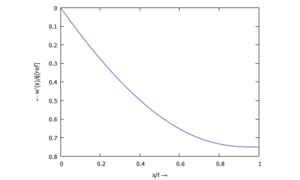
Querschnitts-Kippung w'(x) - ... für M(ξ):
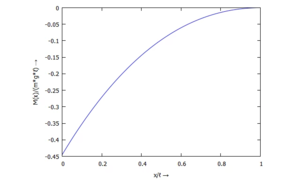
Momentenverlauf M(x) - ... für Q(ξ):
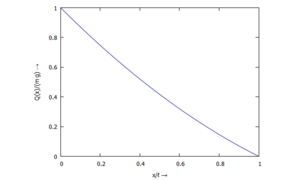
Querkraftverlauf Q(x)
/******************************************************/
/* post-processing */
/* bearing forces and moments */
reactForces: [M[A] = M[ref]*M(0),
Q[z] = Q[ref]*Q(0)];
reactForces: ratsimp(subst(sol, subst(subst([xi=0],displ),subst(reference,reactForces))));
/* plot displacements */
fcts: [ w (xi),
ϕ (xi),
M (xi),
Q (xi)];
textlabels : ["← w(x)/w[rez]", "← w'(x)/ϕ[ref]", "M(x)/(m*g*ℓ) →", "Q(x)/(m g) →"];
for i: 1 thru 4 do(
f : ratsimp(subst(geometry,subst(sol, subst(geometry,subst(dimless,subst(displ,subst(params,fcts[i]))))))),
preamble: if i<=2 then "set yrange [] reverse" else "set yrange []",
plot2d(f, [xi,0,1], [legend, false],
[gnuplot_preamble, preamble],
[xlabel, "x/ℓ →"],
[ylabel, textlabels[i]]) )$
/******************************************************/
/* print tabular values */
for i: 1 thru 4 do(
f : ratsimp(subst(geometry,subst(sol, subst(geometry,subst(dimless,subst(displ,subst(params,fcts[i])))))*facts[i])),
N :100,
print("table for",textlabels[i]),
for j: 0 thru N do (
t : j/N,
print(float(t),";",expand(float(subst([xi=t],f))))
))$
Plot Data
Datenpunkte der Auslenkung w(ξ)
Die Tabelle enthält die Werte ξ und w(ξ) zum Herunterladen.

toggle: data listing →
table for ← w(x)/w[rez]
0.0 ; 0.0
0.01 ; 7.481203031248985*10^-5
0.02 ; 2.984924700020887*10^-4
0.03 ; 6.698993034749879*10^-4
0.04 ; 0.001187879040283986
0.05 ; 0.00185126660292937
0.06 ; 0.002658885214942354
0.07 ; 0.003609546289359847
0.08 ; 0.004702049317703514
0.09 ; 0.005935181759589655
0.1 ; 0.007307718933097404
0.11 ; 0.008818423906038287
0.12 ; 0.01046604738827592
0.13 ; 0.01224932762526032
0.14 ; 0.01416699029293324
0.15 ; 0.01621774839421548
0.16 ; 0.01840030215723649
0.17 ; 0.02071333893554073
0.18 ; 0.02315553311047674
0.19 ; 0.02572554599601331
0.2 ; 0.02842202574623001
0.21 ; 0.03124360726574977
0.22 ; 0.03418891212340282
0.23 ; 0.0372565484694203
0.24 ; 0.04044511095649053
0.25 ; 0.04375318066501066
0.26 ; 0.04717932503291399
0.27 ; 0.05072209779045508
0.28 ; 0.0543800389003753
0.29 ; 0.05815167450388911
0.3 ; 0.06203551687296655
0.31 ; 0.06603006436941357
0.32 ; 0.07013380141128575
0.33 ; 0.07434519844720817
0.34 ; 0.07866271193920962
0.35 ; 0.08308478435471277
0.36 ; 0.08760984416838222
0.37 ; 0.09223630587454254
0.38 ; 0.09696257001097904
0.39 ; 0.1017870231949183
0.4 ; 0.1067080381721125
0.41 ; 0.1117239738799426
0.42 ; 0.1168331755255615
0.43 ; 0.1220339746801493
0.44 ; 0.1273246893904267
0.45 ; 0.1327036243086318
0.46 ; 0.1381690708422802
0.47 ; 0.1437193073250795
0.48 ; 0.1493525992104727
0.49 ; 0.1550671992894059
0.5 ; 0.160861347933972
0.51 ; 0.1667332733687484
0.52 ; 0.1726811919717323
0.53 ; 0.1787033086069086
0.54 ; 0.1847978169906433
0.55 ; 0.1909629000942185
0.56 ; 0.1971967305850093
0.57 ; 0.2034974713089322
0.58 ; 0.2098632758170441
0.59 ; 0.2162922889392689
0.6 ; 0.2227826474085497
0.61 ; 0.229332480538859
0.62 ; 0.2359399109607717
0.63 ; 0.2426030554186048
0.64 ; 0.2493200256333157
0.65 ; 0.2560889292357581
0.66 ; 0.2629078707751271
0.67 ; 0.2697749528078152
0.68 ; 0.2766882770722823
0.69 ; 0.2836459457558966
0.7 ; 0.2906460628602184
0.71 ; 0.2976867356715562
0.72 ; 0.3047660763442248
0.73 ; 0.3118822036044391
0.74 ; 0.3190332445833526
0.75 ; 0.3262173367883804
0.76 ; 0.3334326302226785
0.77 ; 0.340677289663329
0.78 ; 0.3479494971096025
0.79 ; 0.3552474544135462
0.8 ; 0.3625693861060849
0.81 ; 0.3699135424327804
0.82 ; 0.3772782026145832
0.83 ; 0.3846616783500371
0.84 ; 0.392062317576676
0.85 ; 0.3994785085108331
0.86 ; 0.4069086839865492
0.87 ; 0.4143513261159026
0.88 ; 0.4218049712949479
0.89 ; 0.4292682155813812
0.9 ; 0.4367397204721419
0.91 ; 0.4442182191116147
0.92 ; 0.4517025229634247
0.93 ; 0.4591915289818366
0.94 ; 0.4666842273215563
0.95 ; 0.4741797096282359
0.96 ; 0.4816771779554077
0.97 ; 0.4891759543576916
0.98 ; 0.4966754912143446
0.99 ; 0.5041753823419752
1.0 ; 0.5116753749604923
Datenpunkte des Querschnitt-Kippwinkels ϕ(ξ)
Die Tabelle enthält die Werte ξ und ϕ(ξ) zum Herunterladen.

toggle: data listing →
table for ← w'(x)/ϕ[ref]
0.0 ; 0.0
0.01 ; 0.01494356203126184
0.02 ; 0.02977349250076523
0.03 ; 0.04448864954005514
0.04 ; 0.05908788004997918
0.05 ; 0.07357001972386588
0.06 ; 0.08793389308109258
0.07 ; 0.1021783135117721
0.08 ; 0.1163020833333333
0.09 ; 0.1303039938598174
0.1 ; 0.1441828254847645
0.11 ; 0.1579373477786176
0.12 ; 0.1715663196016297
0.13 ; 0.185068489233321
0.14 ; 0.1984425945195976
0.15 ; 0.2116873630387144
0.16 ; 0.2248015122873346
0.17 ; 0.2377837498880229
0.18 ; 0.250632773819587
0.19 ; 0.2633472726717744
0.2 ; 0.2759259259259259
0.21 ; 0.2883674042632877
0.22 ; 0.3006703699027901
0.23 ; 0.3128334769702193
0.24 ; 0.3248553719008265
0.25 ; 0.336734693877551
0.26 ; 0.348470075307174
0.27 ; 0.3600601423368639
0.28 ; 0.3715035154137372
0.29 ; 0.3827988098902226
0.3 ; 0.3939446366782007
0.31 ; 0.4049396029550786
0.32 ; 0.4157823129251701
0.33 ; 0.4264713686399656
0.34 ; 0.4370053708811148
0.35 ; 0.4473829201101928
0.36 ; 0.4576026174895895
0.37 ; 0.4676630659791486
0.38 ; 0.4775628715134888
0.39 ; 0.4873006442652675
0.4 ; 0.496875
0.41 ; 0.5062845615284206
0.42 ; 0.5155279602627784
0.43 ; 0.5246038378838899
0.44 ; 0.5335108481262327
0.45 ; 0.5422476586888658
0.46 ; 0.5508129532804857
0.47 ; 0.55920543380751
0.48 ; 0.5674238227146814
0.49 ; 0.5754668654883558
0.5 ; 0.5833333333333334
0.51 ; 0.5910220260348633
0.52 ; 0.5985317750182615
0.53 ; 0.6058614466194641
0.54 ; 0.6130099455807844
0.55 ; 0.6199762187871581
0.56 ; 0.6267592592592592
0.57 ; 0.6333581104210475
0.58 ; 0.6397718706605832
0.59 ; 0.6459996982043157
0.6 ; 0.6520408163265307
0.61 ; 0.6578945189172403
0.62 ; 0.6635601764335224
0.63 ; 0.6690372422611753
0.64 ; 0.674325259515571
0.65 ; 0.6794238683127573
0.66 ; 0.6843328135442192
0.67 ; 0.6890519531912488
0.68 ; 0.6935812672176308
0.69 ; 0.6979208670823379
0.7 ; 0.7020710059171598
0.71 ; 0.7060320894177032
0.72 ; 0.7098046875
0.73 ; 0.7133895467790936
0.74 ; 0.716787603930461
0.75 ; 0.72
0.76 ; 0.7230280957336108
0.77 ; 0.7258734880031728
0.78 ; 0.728538027411986
0.79 ; 0.7310238371695923
0.8 ; 0.7333333333333333
0.81 ; 0.7354692465221383
0.82 ; 0.7374346452168917
0.83 ; 0.7392329607714223
0.84 ; 0.7408680142687277
0.85 ; 0.7423440453686201
0.86 ; 0.7436657433056325
0.87 ; 0.7448382802098833
0.88 ; 0.7458673469387755
0.89 ; 0.7467591916240565
0.9 ; 0.7475206611570248
0.91 ; 0.748159245854726
0.92 ; 0.7486831275720165
0.93 ; 0.7491012315486069
0.94 ; 0.7494232823068707
0.95 ; 0.7496598639455783
0.96 ; 0.7498224852071006
0.97 ; 0.7499236497313602
0.98 ; 0.7499769319492503
0.99 ; 0.7499970591118518
1.0 ; 0.75
Datenpunkte des Biegemoments M(ξ)
Die Tabelle enthält die Werte ξ und M(ξ) zum Herunterladen.

toggle: data listing →
table for M(x)/(m*g*ℓ) ->
0.0 ; -0.4444444444444444
0.01 ; -0.434511
0.02 ; -0.4247102222222222
0.03 ; -0.4150414444444445
0.04 ; -0.405504
0.05 ; -0.3960972222222222
0.06 ; -0.3868204444444445
0.07 ; -0.377673
0.08 ; -0.3686542222222222
0.09 ; -0.3597634444444445
0.1 ; -0.351
0.11 ; -0.3423632222222222
0.12 ; -0.3338524444444445
0.13 ; -0.325467
0.14 ; -0.3172062222222222
0.15 ; -0.3090694444444445
0.16 ; -0.301056
0.17 ; -0.2931652222222222
0.18 ; -0.2853964444444445
0.19 ; -0.277749
0.2 ; -0.2702222222222222
0.21 ; -0.2628154444444444
0.22 ; -0.255528
0.23 ; -0.2483592222222222
0.24 ; -0.2413084444444444
0.25 ; -0.234375
0.26 ; -0.2275582222222222
0.27 ; -0.2208574444444444
0.28 ; -0.214272
0.29 ; -0.2078012222222222
0.3 ; -0.2014444444444445
0.31 ; -0.195201
0.32 ; -0.1890702222222222
0.33 ; -0.1830514444444444
0.34 ; -0.177144
0.35 ; -0.1713472222222222
0.36 ; -0.1656604444444444
0.37 ; -0.160083
0.38 ; -0.1546142222222222
0.39 ; -0.1492534444444444
0.4 ; -0.144
0.41 ; -0.1388532222222222
0.42 ; -0.1338124444444445
0.43 ; -0.128877
0.44 ; -0.1240462222222222
0.45 ; -0.1193194444444445
0.46 ; -0.114696
0.47 ; -0.1101752222222222
0.48 ; -0.1057564444444444
0.49 ; -0.101439
0.5 ; -0.09722222222222222
0.51 ; -0.09310544444444445
0.52 ; -0.089088
0.53 ; -0.08516922222222222
0.54 ; -0.08134844444444445
0.55 ; -0.077625
0.56 ; -0.07399822222222223
0.57 ; -0.07046744444444444
0.58 ; -0.067032
0.59 ; -0.06369122222222222
0.6 ; -0.06044444444444445
0.61 ; -0.057291
0.62 ; -0.05423022222222222
0.63 ; -0.05126144444444444
0.64 ; -0.048384
0.65 ; -0.04559722222222222
0.66 ; -0.04290044444444444
0.67 ; -0.040293
0.68 ; -0.03777422222222222
0.69 ; -0.03534344444444444
0.7 ; -0.033
0.71 ; -0.03074322222222222
0.72 ; -0.02857244444444445
0.73 ; -0.026487
0.74 ; -0.02448622222222222
0.75 ; -0.02256944444444444
0.76 ; -0.020736
0.77 ; -0.01898522222222222
0.78 ; -0.01731644444444444
0.79 ; -0.015729
0.8 ; -0.01422222222222222
0.81 ; -0.01279544444444444
0.82 ; -0.011448
0.83 ; -0.01017922222222222
0.84 ; -0.008988444444444445
0.85 ; -0.007875
0.86 ; -0.006838222222222222
0.87 ; -0.005877444444444445
0.88 ; -0.004992
0.89 ; -0.004181222222222222
0.9 ; -0.003444444444444444
0.91 ; -0.002781
0.92 ; -0.002190222222222222
0.93 ; -0.001671444444444444
0.94 ; -0.001224
0.95 ; -8.472222222222222*10^-4
0.96 ; -5.404444444444444*10^-4
0.97 ; -3.03*10^-4
0.98 ; -1.342222222222222*10^-4
0.99 ; -3.344444444444444*10^-5
1.0 ; 0.0
Datenpunkte der Querkraft Q(ξ)
Die Tabelle enthält die Werte ξ und Q(ξ) zum Herunterladen.

toggle: data listing →
table for Q(x)/(m g) ->
0.0 ; 1.0
0.01 ; 0.9867
0.02 ; 0.9734666666666667
0.03 ; 0.9603
0.04 ; 0.9472
0.05 ; 0.9341666666666667
0.06 ; 0.9212
0.07 ; 0.9083
0.08 ; 0.8954666666666666
0.09 ; 0.8827
0.1 ; 0.87
0.11 ; 0.8573666666666667
0.12 ; 0.8448
0.13 ; 0.8323
0.14 ; 0.8198666666666666
0.15 ; 0.8075
0.16 ; 0.7952
0.17 ; 0.7829666666666667
0.18 ; 0.7708
0.19 ; 0.7587
0.2 ; 0.7466666666666667
0.21 ; 0.7347
0.22 ; 0.7228
0.23 ; 0.7109666666666666
0.24 ; 0.6992
0.25 ; 0.6875
0.26 ; 0.6758666666666666
0.27 ; 0.6643
0.28 ; 0.6528
0.29 ; 0.6413666666666666
0.3 ; 0.63
0.31 ; 0.6187
0.32 ; 0.6074666666666667
0.33 ; 0.5963
0.34 ; 0.5852
0.35 ; 0.5741666666666667
0.36 ; 0.5632
0.37 ; 0.5523
0.38 ; 0.5414666666666667
0.39 ; 0.5307
0.4 ; 0.52
0.41 ; 0.5093666666666666
0.42 ; 0.4988
0.43 ; 0.4883
0.44 ; 0.4778666666666667
0.45 ; 0.4675
0.46 ; 0.4572
0.47 ; 0.4469666666666667
0.48 ; 0.4368
0.49 ; 0.4267
0.5 ; 0.4166666666666667
0.51 ; 0.4067
0.52 ; 0.3968
0.53 ; 0.3869666666666667
0.54 ; 0.3772
0.55 ; 0.3675
0.56 ; 0.3578666666666667
0.57 ; 0.3483
0.58 ; 0.3388
0.59 ; 0.3293666666666666
0.6 ; 0.32
0.61 ; 0.3107
0.62 ; 0.3014666666666667
0.63 ; 0.2923
0.64 ; 0.2832
0.65 ; 0.2741666666666667
0.66 ; 0.2652
0.67 ; 0.2563
0.68 ; 0.2474666666666667
0.69 ; 0.2387
0.7 ; 0.23
0.71 ; 0.2213666666666667
0.72 ; 0.2128
0.73 ; 0.2043
0.74 ; 0.1958666666666667
0.75 ; 0.1875
0.76 ; 0.1792
0.77 ; 0.1709666666666667
0.78 ; 0.1628
0.79 ; 0.1547
0.8 ; 0.1466666666666667
0.81 ; 0.1387
0.82 ; 0.1308
0.83 ; 0.1229666666666667
0.84 ; 0.1152
0.85 ; 0.1075
0.86 ; 0.09986666666666667
0.87 ; 0.0923
0.88 ; 0.0848
0.89 ; 0.07736666666666667
0.9 ; 0.07
0.91 ; 0.0627
0.92 ; 0.05546666666666666
0.93 ; 0.0483
0.94 ; 0.0412
0.95 ; 0.03416666666666666
0.96 ; 0.0272
0.97 ; 0.0203
0.98 ; 0.01346666666666667
0.99 ; 0.0067
1.0 ; 0.0
Links
Literature
- ...