Gelöste Aufgaben/JUMP/Car-Body

← Back to Start

Scope

Car-Body

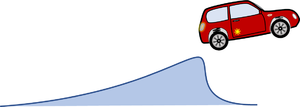
In common simulation applications - especially for full-size commercial cars - the pitch-angle of the car is assumed to be small to allow for a linearization of geometry and most parts of the equations of motion. We drop this limitation so we can do more fancy stuff with our model - like climbing steep roads or jumping across ditches.

Block Diagram.

We employ a spatial x-y coordinate system, x in horizontal, y in vertical, upwards direction. And we’ll briefly employ the z-axis as rotation direction - which is towards you following the “right-hand-rule“ for coordinate systems.

Structure

The driver controls the car's motion via the position of the "gas"-pedal, which is being translated into a torque M_W at the wheel.

This toque will change velocities and thus the position of the car. These state-variables will then create - via info - a feedback to the driver.

We’ll need to invest significant efforts in describing the kinematics of the car-motion and to derive its equations of body-motion since we do not want to limit our study on small pitch-angles of the car. Key accessory will be vectors, which map locations like the center of mass:

${\vec {r}}_{C}(t)$.

This vector has - in 2D - two coordinates $u_{1}(t),u_{2}(t)$, measured in the inertial x-y frame:

${\vec {r}}_{C}(t)={\vec {\underline {e}}}_{0}\cdot \left({\begin{array}{c}u_{1}\\u_{2}\end{array}}\right)$ with ${\vec {\underline {e}}}_{0}=\left({\vec {e}}_{x},{\vec {e}}_{y}\right)$.

representing the unit vectors spanning the x-y space. If we refer to a specific frame, we may drop the vector-notation $({\vec {.}})$ and refer to the column-matrix of coordinates $({\underline {.}})$ only, so

${\underline {r}}_{C}=\left({\begin{array}{c}u_{1}\\u_{2}\end{array}}\right)$.

We’ll also employ coordinate transformations using Euler-rotations.

The car with front-wheel drive consists of the car-body with center of mass “C“, the front wheels “A“ and the rear wheel “B“. Masses of car-body and wheel-sets are M and m respectively.The geometry of the car is described by

• a₀: the wheel base;
• a₁: longitudinal distance between center of mass and front-wheel-hub;
• a₂ = a₀ - a₁ and
• a₃: vertical distance between center of mass and front-wheel-hub (relaxed springs).

tmp

Body

We use five coordinates to describe the motion of the car:

• $u_{1}(t),u_{2}(t),\varphi (t)$ for the location of the center of mass of the car-body and its pitch-angle
  ,
• $u_{3},u_{4}$ for the "vertical" motion of the wheel hubs relative to the car-body - which is synonym to the compression of the springs
  
  and
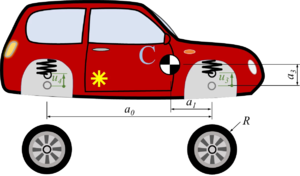
• $\psi (t)$ as the rotation angle of the front-wheel - we'll not account for the rotation of the rear-wheel.

So the center of mass of the car-body is

${\vec {r}}_{M}={\vec {\underline {e}}}_{0}\cdot \left({\begin{array}{c}u_{1}(t)\\u_{2}(t)\end{array}}\right)$,

the coordinate system of the car is

${\vec {\underline {e}}}_{C}={\vec {\underline {e}}}_{0}\cdot {\underline {\underline {D}}}(\varphi (t))$

where

${\underline {\underline {D}}}(\varphi )=\left({\begin{array}{rr}\cos(\varphi )&-\sin(\varphi )\\+\sin(\varphi )&\cos(\varphi )\\\end{array}}\right)$.

So the location of the front-wheel “A“ is

${\vec {r}}_{A}={\vec {r}}_{M}+{\vec {r}}_{MA}$

or

${\underline {r}}_{A}={\underline {r}}_{M}+{\underline {\underline {D}}}(\varphi (t))\cdot \left({\begin{array}{c}a_{1}\\-a_{3}+u_{3}(t)\end{array}}\right)$.

Likewise, the location of the rear-wheel “B“ is

${\underline {r}}_{B}={\underline {r}}_{M}+{\underline {\underline {D}}}(\varphi (t))\cdot \left({\begin{array}{c}-a_{2}\\-a_{3}+u_{4}(t)\end{array}}\right)$

and in the following, we’ll be using the abbreviations

$\left({\begin{array}{c}U_{3}\\U_{4}\end{array}}\right)=:{\underline {r}}_{A}$

and

$\left({\begin{array}{c}U_{5}\\U_{6}\end{array}}\right)=:{\underline {r}}_{B}$.

tmp

Track

Tack specification

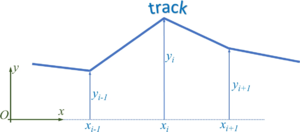
We describe the road by road-sections, which we call tracks. We start by defining each track as a line-segment, i.e. a first-order polynomial. The road is thus defined by pairs of track-endpoints, i.e. (x_i, y_i).

The track-polynomials are

${\begin{array}{ll}t_{i}(x)&=y_{i}\cdot {\tilde {x}}_{i}+y_{i-1}\cdot \left(1-{\tilde {x}}_{i}\right){\text{ for }}{\tilde {x}}_{i}={\frac {\displaystyle x-x_{i-1}}{\displaystyle x_{i}-x_{i-1}}}\\&=T_{i,1}\cdot x+T_{i,0}\end{array}}$.

During the numerical integration of the initial-value-problem - when the car runs along the track - the solver needs to cope with the transition between two straight lines - which is numerically more efficient if we convert the road in a continuously differentiable function.

Rounding the edges.

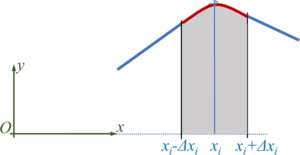
For this purpose, we define transition polynomials p_i of second degree - parabolas - as

$p_{i}(x)=\displaystyle \sum _{j=0}^{2}P_{i,j}\cdot x^{j}$

which connect two neighboring tracks.

The boundary conditions for the parabolas (red) and the neighboring lines (blue) yield

${\begin{array}{ll}\color {blue}t_{i}(x_{i}-\Delta x_{i})&=\color {red}p_{i}(x_{i}-\Delta x_{i}),\\\color {blue}\left.{\frac {\displaystyle dt_{i}}{\displaystyle dx_{i}}}\right\vert _{x_{i}-\Delta x_{i}}&=\color {red}\left.{\frac {\displaystyle dp_{i}}{\displaystyle dx_{i}}}\right\vert _{x_{i}-\Delta x_{i}},\\\color {blue}t_{i+1}(x_{i}+\Delta x_{i})&=\color {red}p_{i}(x_{i}+\Delta x_{i}),\\\color {blue}\left.{\frac {\displaystyle dt_{i+1}}{\displaystyle dx_{i+1}}}\right\vert _{x_{i}+\Delta x_{i}}&=\color {red}\left.{\frac {\displaystyle dp_{i}}{\displaystyle dx_{i}}}\right\vert _{x_{i}+\Delta x_{i}}.\end{array}}$

And though we have four boundary conditions, they allow for a solution with three unknown polynomial coefficients P_i if Δx_i is identical left and right from x_i - which we have already implied.

${\begin{array}{ll}{b_{3}}&=0,\\{b_{2}}&={\frac {\displaystyle {a_{2,1}}-{a_{1,1}}}{\displaystyle 4{\mathit {\Delta x_{i}}}}},\\{b_{1}}&={\frac {\displaystyle \left({a_{2,1}}+{a_{1,1}}\right)\,{\mathit {\Delta x_{i}}}-{x_{i}}\,{a_{2,1}}+{x_{i}}\,{a_{1,1}}}{\displaystyle 2{\mathit {\Delta x_{i}}}}},\\{b_{0}}&={\frac {\displaystyle \left({a_{2,1}}-{a_{1,1}}\right)\,{{\mathit {\Delta x_{i}}}^{2}}+\left(-2{x_{i}}\,{a_{2,1}}-2{x_{1}}\,{a_{1,1}}+4{y_{i}}\right)\,{\mathit {\Delta x_{i}}}+{{x}_{i}^{2}}\,{a_{2,1}}-{{x}_{i}^{2}}\,{a_{1,1}}}{\displaystyle 4{\mathit {\Delta x_{i}}}}}\end{array}}$

and

${\begin{array}{ll}{a_{1,0}}&={y_{i}}-{x_{i}}\,{a_{1,1}},\\{a_{1,1}}&={\frac {\displaystyle {y_{i-1}}-{y_{i}}}{\displaystyle {x_{i-1}}-{x_{i}}}}\operatorname {,} \\{a_{2,0}}&={y_{i}}-{x_{i}}\,{a_{2,1}},\\{a_{2,1}}&={\frac {\displaystyle {y_{i}}-{y_{i+1}}}{\displaystyle {x_{i}}-{x_{i+1}}}}\end{array}}$

But instead of employing the above, we solve the linear systems of equations for the coefficients numerically.

Now each polynomial creates new endpoints x_i - Δx_i and x_i + Δx_i which substitute for the initial x_i-point. The tracks are represented by a succession of lines and parabolas.

Next we need to find the contact point between wheel and road!

/*******************************************************/
/* MAXIMA script                                       */
/* version: wxMaxima 16.04.2                           */
/* author: Andreas Baumgart                            */
/* last updated: 2021-02-08                            */
/* ref: Modelling and Simulation (TUAS)                */
/* description: Rounding between straight line-segments*/
/*******************************************************/

/*******************************************************/
/* declarations                                        */
/*******************************************************/
declare("Δ",alphabetic);

params: [x[0] = 0, x[1] = 10, x[2]=15,
         y[0] = 0, y[1] =  1, y[2]=-1,
	 eps = 0.2];

/*******************************************************/
/* polynomials                                         */
/*******************************************************/
/* polynomial coefficiets of two consecutive straigt lines */
P : [[a[1,1],a[1,0]],[a[2,1],a[2,0]]];
/* polynomials of cubic and two consecutive linear polynomials */
p : [a[1,1]*x+a[1,0],
     b[3]*x^3+b[2]*x^2+b[1]*x+b[0],
     a[2,1]*x+a[2,0]];

/* compute polynomial coefficients from (x[i]/y[i])-points*/
linear : solve([subst([x=x[0]],p[1]) = y[0],
                subst([x=x[1]],p[1]) = y[1],
		subst([x=x[1]],p[3]) = y[1],
		subst([x=x[2]],p[3]) = y[2]], [a[1,0],a[1,1],a[2,0],a[2,1]])[1];
/* equations to continuously fit cubic function to lines */
equs : [subst([x=x[1]-Δx], p[1]) = subst([x=x[1]-Δx], p[2]),
        subst([x=x[1]+Δx], p[3]) = subst([x=x[1]+Δx], p[2]),
	subst([x=x[1]-Δx], diff(p[1],x)) = subst([x=x[1]-Δx], diff(p[2],x)),
	subst([x=x[1]+Δx], diff(p[3],x)) = subst([x=x[1]+Δx], diff(p[2],x))];
/* unknowns */
Q : [b[3],b[2],b[1],b[0]];

/* solve for unknowns */
sol : ratsimp(subst(linear,solve(equs,Q)[1]));

/* solve linear system of equations for unknown coefficients */
ACM : augcoefmatrix(equs,Q);
print(submatrix(ACM,5)," * ",transpose(Q)," = ",col(ACM,5))$


/* or - more simple when doing it numerically  .... */

/* coefficients of straigt line polynomials */
s : makelist(a[i,1] = (y[i-1]-y[i])/(x[i-1]-x[i]),i,1,2);

P : [[a[1,1],a[1,0]],[a[2,1],a[2,0]]];
p : [a[1,1]*x+a[1,0],
     b[3]*x^3+b[2]*x^2+b[1]*x+b[0],
     a[2,1]*x+a[2,0]];

linear : solve([subst([x=x[1]],p[1]) = y[1],
		subst([x=x[1]],p[3]) = y[1]], [a[1,0],a[2,0]])[1];

equs : [subst([x=x[1]-Δx], p[1]) = subst([x=x[1]-Δx], p[2]),
        subst([x=x[1]+Δx], p[3]) = subst([x=x[1]+Δx], p[2]),
	subst([x=x[1]-Δx], diff(p[1],x)) = subst([x=x[1]-Δx], diff(p[2],x)),
	subst([x=x[1]+Δx], diff(p[3],x)) = subst([x=x[1]+Δx], diff(p[2],x))];
Q : [b[3],b[2],b[1],b[0]];

sol : ratsimp(subst(linear,solve(equs,Q)))[1];

/* check with parameters */
subst(params,subst(s,subst(linear,solve(equs,Q))));

tmp

Contact-Point and -Normal

Contact conditions.

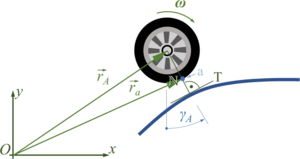
Finding the contact between wheel and road is our next challenge. We need to differentiate between the straight lines and the parabolas - but in both cases, we are looking for the equation of the normal “N“ to the road-surface, which cuts through the wheel-hub.

From the car-coordinates, we know the location of the wheel - e.g. “A” - expressed by its hub-vector

${\vec {r}}_{A}={\vec {\underline {e}}}_{0}\cdot \left({\begin{array}{c}U_{3}(t)\\U_{4}(t)\end{array}}\right)$.

And we can also define

${\vec {r}}_{A}(t)={\vec {r}}_{a}(t)+{\vec {N}}_{A}(t)$.

where ${\vec {r}}_{A}$ is the vector to the contact point “a“ of wheel “A“ and ${\vec {N}}_{A}$ is the vector from “a“ to the wheel hub “A“. Since "a" is a point on a track - if wheel and road have contact - and the direction of N_A is known to be perpendicular to this track in “a”, we have two equations for the two unknowns “point on track“ and “length of normal ${\vec {N}}_{A}$“.

Contact angles

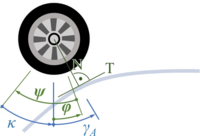
However, it remains tricky to find the material coordinate of the wheel that is at “a“. The picture below explains how first rotate the car by φ - positive about the z-axis, then rotate the wheel in negative direction by ψ and by κ in positive direction back to vertical. From there it is the known angle γ_A to “a“.

This succession of rotations is expressed by

$\gamma _{A}(t)=\varphi (t)-\psi (t)+\kappa (t)$

and we find contact point “a“ from

${\begin{array}{ll}{\underline {r}}_{a}&={\underline {r}}_{A}+{\underline {\underline {D}}}(\gamma _{A}(t))\cdot \left({\begin{array}{c}0\\-R\end{array}}\right)\\&={\begin{pmatrix}+R\sin {\left(\operatorname {\gamma } _{A}(t)\right)}-\left({u_{3}}(t)-{a_{3}}\right)\sin {\left(\operatorname {\varphi } (t)\right)}+{a_{1}}\cos {\left(\operatorname {\varphi } (t)\right)}+{u_{1}}(t)\\-R\cos {\left(\operatorname {\gamma } _{A}(t)\right)}+{a_{1}}\sin {\left(\operatorname {\varphi } (t)\right)}+\left({u_{3}}(t)-{a_{3}}\right)\cos {\left(\operatorname {\varphi } (t)\right)}+{u_{2}}(t)\end{pmatrix}}\end{array}}$

and “b“ from

${\begin{array}{ll}{\underline {r}}_{b}&={\underline {r}}_{B}+{\underline {\underline {D}}}(\gamma _{B}(t))\cdot \left({\begin{array}{c}0\\-R\end{array}}\right)\\&={\begin{pmatrix}+\sin {\left({\gamma _{B}}\right)}R-\left({u_{4}}(t)-{a_{3}}\right)\sin {\left(\operatorname {\varphi } (t)\right)}-{a_{2}}\cos {\left(\operatorname {\varphi } (t)\right)}+{u_{1}}(t)\\-\cos {\left({\gamma _{B}}\right)}R-{a_{2}}\sin {\left(\operatorname {\varphi } (t)\right)}+\left({u_{4}}(t)-{a_{3}}\right)\cos {\left(\operatorname {\varphi } (t)\right)}+{u_{2}}(t)\end{pmatrix}}\end{array}}$.

For wheel A und B, the angles ψ and κ are not identical - but we have dropped the indices here for brevity.

Track is a Line

If the track is line “i“, we have

${\vec {r}}_{a}={\vec {\underline {e}}}_{0}\cdot \left[\right.\underbrace {\left({\begin{array}{c}x_{i-1}\\y_{i-1}\end{array}}\right)} _{\displaystyle :={\underline {P}}_{i-1}}+\xi \cdot \underbrace {\left({\begin{array}{c}x_{i}-x_{i-1}\\y_{i}-y_{i-1}\end{array}}\right)} _{:=\displaystyle {\underline {\Delta P}}_{i}}\left.\right]$

with

${\underline {P}}_{i-1},{\underline {P}}_{i}$

being start- end end-points of the line with coordinates [x_i, h_i] and ξ naming the coordinate of point “C“. For the track-normal, we have

${\vec {N}}_{A}=\eta \cdot R\cdot {\vec {n}}_{A}$

with the unit-normal , the wheel-radius R and the unknown η. For the unit-normal

${\vec {N}}_{A}\cdot \Delta {\vec {P}}_{i}=0{\text{ and }}{\vec {n}}_{A}\cdot {\vec {n}}_{A}=1$

holds.

And for the contact angles γ_A/B we employ

$\tan \gamma _{A}(t)={\frac {\displaystyle N_{A,y}}{\displaystyle N_{A,x}}}$.

Track is a Parabola

If the track is defined by a second-order polynomial p_i, we proceed accordingly. However, the track inclination is linearly dependent on “x” and we find that the normal is proportional to:

${\underline {\tilde {N}}}=\left({\begin{array}{c}1\\-{\frac {\displaystyle 1}{\displaystyle 2\;P_{i,2}\;x+P_{i,1}}}\end{array}}\right).$

From

${\underline {r}}_{A}=\left({\begin{array}{c}x\\p_{i}(x)\end{array}}\right)+\eta \cdot {\underline {\tilde {N}}}{\text{ with }}{\underline {r}}_{A}=\left({\begin{array}{c}U_{3}\\U_{4}\end{array}}\right)$

we get two equations for the unknowns “x“ and “η“ as

${\begin{array}{ll}u_{3}&=\eta +x,\\u_{4}&=-{\frac {\displaystyle \eta }{\displaystyle 2P_{i,2}\;x+P_{i,1}}}+P_{i,2}\,{{x}^{2}}+P_{i,1}x+P_{i,0}\end{array}}$.

And we get a third-degree polynomial in “x“ from the second equation. The three solutions for point “C” usually comprise complex (they have a real and imaginary part) solutions depending on the curvature (convex or concave) of the parabola. That’s not a problem but increases computational cost significantly - because we will need to solve this equation for each value of U₃, U₄ again and again.

tmp

Contact Forces

Wheel kinematics

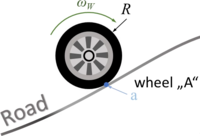
At contact “a” between road and wheel, the motor-torque must be transformed into a propulsion force of the car.

In the freebody-diagram for wheel “A“ below, we account for the contact forces

• normal force N_a and
• traction force F.

The interaction between road and wheel is a complex relationship between these two forces and the relative velocity v_rel of the wheel in point C relative to the road.

Contact forces F, N

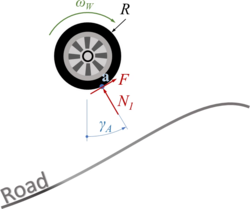
For the normal force N_A we assume a characteristic which depends on the dimensionless compression of the wheel e = 1-η, where - obviously -

$N_{a}=\left\{{\begin{array}{l}0{\text{ for }}e<0\\{\text{'positive' for }}e>0\end{array}}\right.$

To have a continuously differentiable function N_A as in this contact-characteristic, we choose a parabola for 0<e<ε with continuous transitions to

• e=0 for e<0 and
• e=k_C η for e>ε:
  

  

  Characteristic for contact force.

And - for the sake of simplicity - let

$N_{a}(\varepsilon )=m\cdot g$.

Now, for the traction force, we assume

${\begin{array}{ll}F&=F(N_{a},v_{rel})\\&=\mu (v_{rel})\cdot N_{a}\end{array}}$

and introduce a point-symmetric characteristic for this relationship μ(v_rel):

Friction characteristic

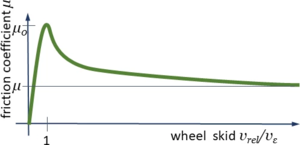
It mimics the key properties of dry friction with a stick-coefficient μ₀ for very low relative velocities and μ else. To simplify implementation, we’ll be using the same mechanism as for the tracks to compose this characteristic from lines and transition parabolas.

1+1=2

tmp

Track

Text

1+1=2

tmp

Contact Forces

Text

1+1=2

tmp

Track

Text

1+1=2

tmp

Track

Text

1+1=2

tmp

Track

Text

1+1=2

tmp

Track

Text

1+1=2

Parameter

x

y

z

a

b

c

next workpackage: driver-controls →

---

References

• ...