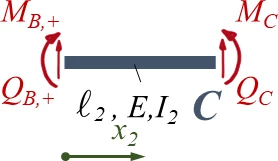Gelöste Aufgaben/TC12: Unterschied zwischen den Versionen
| Zeile 106: | Zeile 106: | ||
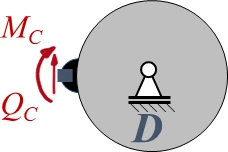
====Aus Rand "A"==== | ====Aus Rand "A"==== | ||
<table class="wikitable" style="background-color:white;"> | <table class="wikitable" style="background-color:white;"> | ||
<tr><td>[[Datei:TC12-11A.png|rahmenlos|alternativtext=| | <tr><td>[[Datei:TC12-11A.png|rahmenlos|alternativtext=|90x90px]] | ||
</td><td>''Geometrische Randbedingungen'' | </td><td>''Geometrische Randbedingungen'' | ||
# <math>w_1(0)=0</math> | |||
''Kraft- und Momenten-Randbedingungen'' | ''Kraft- und Momenten-Randbedingungen'' | ||
#<math>M_{A} = 0 \text{ mit } M_{A} = - EI_1\cdot w''(x)|_{x=0}</math> | |||
# <math>M_{A | |||
</td></tr> | </td></tr> | ||
</table> | </table> | ||
| Zeile 120: | Zeile 118: | ||
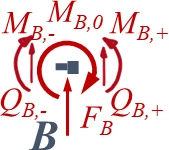
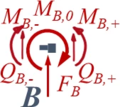
====Aus Übergang "B"==== | ====Aus Übergang "B"==== | ||
<table class="wikitable" style="background-color:white;"> | <table class="wikitable" style="background-color:white;"> | ||
<tr><td>[[Datei:TC12-11B.png|rahmenlos|alternativtext=| | <tr><td>[[Datei:TC12-11B.png|rahmenlos|alternativtext=|120x120px]] | ||
</td><td>''Geometrische Randbedingungen'' | </td><td>''Geometrische Randbedingungen'' | ||
# <math>w_1(\ell_1)=w_2(\ell_1)</math> | # <math>w_1(\ell_1)=w_2(\ell_1)</math> | ||
| Zeile 127: | Zeile 125: | ||
''Kraft- und Momenten-Randbedingungen'' | ''Kraft- und Momenten-Randbedingungen'' | ||
# <math>-M_{B,-} + M_{B,+} = 0</math> | #<math>-M_{B,-} - M_{B0} + M_{B,+} = 0</math> | ||
# <math>-Q_{B,-} - | #<math>-Q_{B,-}-k_B\cdot w_B + -Q_{B,+} = 0</math> | ||
</td></tr> | </td></tr> | ||
</table> | </table> | ||
| Zeile 134: | Zeile 132: | ||
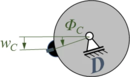
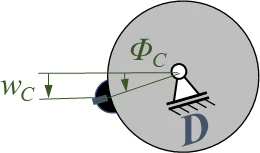
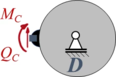
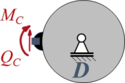
====Aus Rand "C"==== | ====Aus Rand "C"==== | ||
<table class="wikitable" style="background-color:white;"> | <table class="wikitable" style="background-color:white;"> | ||
<tr><td>[[Datei:TC12-11C1.png|rahmenlos|alternativtext=| | <tr><td>[[Datei:TC12-11C1.png|rahmenlos|alternativtext=|125x125px]]<br/> | ||
[[Datei:TC12-11C2.png|rahmenlos|alternativtext=| | [[Datei:TC12-11C2.png|rahmenlos|alternativtext=|130x130px]] | ||
</td><td>''Geometrische Randbedingungen'' | </td><td>''Geometrische Randbedingungen'' | ||
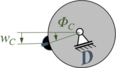
# <math> | #<math>w_C = r\cdot\Phi_C \text{ mit } \Phi_C = - \phi_2(l_2)</math> | ||
''Kraft- und Momenten-Randbedingungen'' | ''Kraft- und Momenten-Randbedingungen'' | ||
# <math> | #<math>r\cdot Q_{C} + M_C = 0</math> | ||
</td></tr> | </td></tr> | ||
</table> | </table> | ||
| Zeile 147: | Zeile 145: | ||
Einsetzen liefert 8 Gleichungen | Einsetzen liefert 8 Gleichungen | ||
<math>\begin{array}{rl} \displaystyle \frac{{{C}_{1,0}}}{{{\mathit{EI}}_{1}}}&=0\ | <math>\begin{array}{rl} \displaystyle \frac{{{C}_{1,0}}}{{{\mathit{EI}}_{1}}}&=0\\ \displaystyle -{{C}_{1,2}}&=0\\ \displaystyle \frac{{{l}_{1}^{3}}\cdot {{C}_{1,3}}}{6\cdot {{\mathit{EI}}_{1}}}+\frac{{{l}_{1}^{2}}\cdot {{C}_{1,2}}}{2\cdot {{\mathit{EI}}_{1}}}+\frac{{{l}_{1}}\cdot {{C}_{1,1}}}{{{\mathit{EI}}_{1}}}+\frac{{{C}_{1,0}}}{{{\mathit{EI}}_{1}}}+\frac{{{q}_{0}}\cdot {{l}_{1}^{4}}}{24\cdot {{\mathit{EI}}_{1}}}&=\frac{{{C}_{2,0}}}{{{\mathit{EI}}_{2}}}\\ \displaystyle \frac{{{l}_{1}^{2}}\cdot {{C}_{1,3}}}{2\cdot {{\mathit{EI}}_{1}}}+\frac{{{l}_{1}}\cdot {{C}_{1,2}}}{{{\mathit{EI}}_{1}}}+\frac{{{C}_{1,1}}}{{{\mathit{EI}}_{1}}}+\frac{{{q}_{0}}\cdot {{l}_{1}^{3}}}{6\cdot {{\mathit{EI}}_{1}}}&=\frac{{{C}_{2,1}}}{{{\mathit{EI}}_{2}}}\\ \displaystyle -\frac{{{C}_{2,0}}\cdot {{k}_{B}}}{{{\mathit{EI}}_{2}}}-{{C}_{2,3}}+{{C}_{1,3}}+{{q}_{0}}\cdot {{l}_{1}}&=0\\ \displaystyle -{{C}_{2,2}}+{{l}_{1}}\cdot {{C}_{1,3}}+{{C}_{1,2}}+\frac{{{q}_{0}}\cdot {{l}_{1}^{2}}}{2}&={{M}_{B}}\\ \displaystyle -\frac{{{l}_{2}^{2}}\cdot {{C}_{2,3}}\cdot r}{2\cdot {{\mathit{EI}}_{2}}}-\frac{{{l}_{2}}\cdot {{C}_{2,2}}\cdot r}{{{\mathit{EI}}_{2}}}-\frac{{{C}_{2,1}}\cdot r}{{{\mathit{EI}}_{2}}}&=\displaystyle\frac{{{l}_{2}^{3}}\cdot {{C}_{2,3}}}{6\cdot {{\mathit{EI}}_{2}}}+\frac{{{l}_{2}^{2}}\cdot {{C}_{2,2}}}{2\cdot {{\mathit{EI}}_{2}}}+\frac{{{l}_{2}}\cdot {{C}_{2,1}}}{{{\mathit{EI}}_{2}}}+\frac{{{C}_{2,0}}}{{{\mathit{EI}}_{2}}}\\ \displaystyle -{{C}_{2,3}}\cdot r-{{l}_{2}}\cdot {{C}_{2,3}}-{{C}_{2,2}}&=0 \end{array} | ||
</math> | |||
für die Integrationskonstanten. | für die Integrationskonstanten. | ||
<!--------------------------------------------------------------------------------> | <!--------------------------------------------------------------------------------> | ||
| Zeile 169: | Zeile 162: | ||
==tmp== | ==tmp== | ||
<!--------------------------------------------------------------------------------> | Das Gleichungssystem wollen wir als | ||
<math>\underline{\underline{A}}\cdot\underline{x}= \underline{b}</math> | |||
schreiben, also | |||
<math>\begin{pmatrix}\frac{1}{{{\mathit{EI}}_{1}}} & 0 & 0 & 0 & 0 & 0 & 0 & 0\\ 0 & 0 & -1 & 0 & 0 & 0 & 0 & 0\\ \frac{1}{{{\mathit{EI}}_{1}}} & \frac{{{\ell}_{1}}}{{{\mathit{EI}}_{1}}} & \frac{{{\ell}_{1}^{2}}}{2\cdot {{\mathit{EI}}_{1}}} & \frac{{{\ell}_{1}^{3}}}{6\cdot {{\mathit{EI}}_{1}}} & -\frac{1}{{{\mathit{EI}}_{2}}} & 0 & 0 & 0\\ 0 & \frac{1}{{{\mathit{EI}}_{1}}} & \frac{{{\ell}_{1}}}{{{\mathit{EI}}_{1}}} & \frac{{{\ell}_{1}^{2}}}{2\cdot {{\mathit{EI}}_{1}}} & 0 & -\frac{1}{{{\mathit{EI}}_{2}}} & 0 & 0\\ 0 & 0 & 0 & 1 & -\frac{{{k}_{B}}}{{{\mathit{EI}}_{2}}} & 0 & 0 & -1\\ 0 & 0 & 1 & {{\ell}_{1}} & 0 & 0 & -1 & 0\\ 0 & 0 & 0 & 0 & -\frac{1}{{{\mathit{EI}}_{2}}} & -\frac{{{\ell}_{2}}+r}{{{\mathit{EI}}_{2}}} & -\frac{{{\ell}_{2}^{2}}+2\cdot {{\ell}_{2}}\cdot r}{2\cdot {{\mathit{EI}}_{2}}} & -\frac{{{\ell}_{2}^{3}}+3\cdot {{\ell}_{2}^{2}}\cdot r}{6\cdot {{\mathit{EI}}_{2}}}\\ 0 & 0 & 0 & 0 & 0 & 0 & -1 & -r-{{\ell}_{2}}\end{pmatrix}\cdot\begin{pmatrix}{{C}_{1,0}}\\ {{C}_{1,1}}\\ {{C}_{1,2}}\\ {{C}_{1,3}}\\ {{C}_{2,0}}\\ {{C}_{2,1}}\\ {{C}_{2,2}}\\ {{C}_{2,3}}\end{pmatrix}=\begin{pmatrix}0\\ 0\\ -\frac{{{q}_{0}}\cdot {{\ell}_{1}^{4}}}{24\cdot {{\mathit{EI}}_{1}}}\\ -\frac{{{q}_{0}}\cdot {{\ell}_{1}^{3}}}{6\cdot {{\mathit{EI}}_{1}}}\\ -{{q}_{0}}\cdot {{\ell}_{1}}\\ {{M}_{B}}-\frac{{{q}_{0}}\cdot {{\ell}_{1}^{2}}}{2}\\ 0\\ 0\end{pmatrix}</math>. | |||
Die Matrix-Elemente sind für die Koeffizientenmatrix | |||
<math>\begin{array}{l} a_{1,1} = 1/EI_{1}\\ a_{2,3} = -1\\ a_{3,1} = 1/EI_{1}\\ a_{3,2} = \ell_{1}/EI_{1}\\ a_{3,3} = \ell_{1}^2/(2\cdot EI_{1})\\ a_{3,4} = \ell_{1}^3/(6\cdot EI_{1})\\ a_{3,5} = -1/EI_{2}\\ a_{4,2} = 1/EI_{1}\\ a_{4,3} = \ell_{1}/EI_{1}\\ a_{4,4} = \ell_{1}^2/(2\cdot EI_{1})\\ a_{4,6} = -1/EI_{2}\\ a_{5,4} = 1\\ a_{5,5} = -k_B/EI_{2}\\ a_{5,8} = -1\\ a_{6,3} = 1\\ a_{6,4} = \ell_{1}\\ a_{6,7} = -1\\ a_{7,5} = -1/EI_{2}\\ a_{7,6} = -(r+\ell_{2})/EI_{2}\\ a_{7,7} = -(2\cdot \ell_{2}\cdot r+\ell_{2}^2)/(2\cdot EI_{2})\\ a_{7,8} = -(3\cdot \ell_{2}^2\cdot r+\ell_{2}^3)/(6\cdot EI_{2})\\ a_{8,7} = -1\\ a_{8,8} = -r-\ell_{2}\\ \end{array}</math> | |||
und für die rechte Seite | |||
<math>\begin{array}{l} b_{1} = 0\\ b_{2} = 0\\ b_{3} = -(q_0\cdot\ell_{1}^4)/(24\cdot EI_{1})\\ b_{4} = -(q_0\cdot\ell_{1}^3)/(6\cdot EI_{1})\\ b_{5} = -q_0\cdot\ell_{1}\\ b_{6} = M_B-(q_0\cdot\ell_{1}^2)/2\\ b_{7} = 0\\ b_{8} = 0 \end{array}</math><!--------------------------------------------------------------------------------> | |||
{{MyCodeBlock|title=Prepare for Solver | {{MyCodeBlock|title=Prepare for Solver | ||
|text=Text | |text=Text | ||
| Zeile 180: | Zeile 189: | ||
==tmp== | ==tmp== | ||
<!--------------------------------------------------------------------------------> | Das Lösen des Gleichungssystems liefert | ||
<math>\begin{array}{ll} \displaystyle {{C}_{1,0}}&\displaystyle =0\\ \displaystyle {{C}_{1,1}}&\displaystyle =-\frac{{{q}_{0}}\cdot {{\ell}_{1}^{3}}}{56}\\ \displaystyle {{C}_{1,2}}&\displaystyle =0\\ \displaystyle {{C}_{1,3}}&\displaystyle =-\frac{{{q}_{0}}\cdot {{\ell}_{1}}}{7}\\ \displaystyle {{C}_{2,0}}&\displaystyle =0\\ \displaystyle {{C}_{2,1}}&\displaystyle =\frac{13\cdot {{q}_{0}}\cdot {{\ell}_{1}^{3}}}{84}\\ \displaystyle {{C}_{2,2}}&\displaystyle =-\frac{9\cdot {{q}_{0}}\cdot {{\ell}_{1}^{2}}}{14}\\ \displaystyle {{C}_{2,3}}&\displaystyle =\frac{6\cdot {{q}_{0}}\cdot {{\ell}_{1}}}{7} \end{array}</math><!--------------------------------------------------------------------------------> | |||
{{MyCodeBlock|title=Solving | {{MyCodeBlock|title=Solving | ||
|text=Text | |text=Text | ||
| Zeile 191: | Zeile 203: | ||
==tmp== | ==tmp== | ||
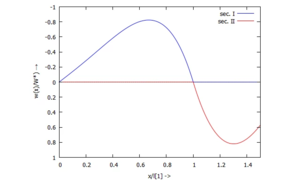
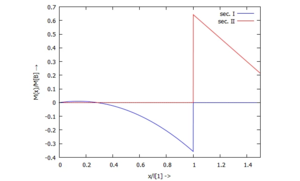
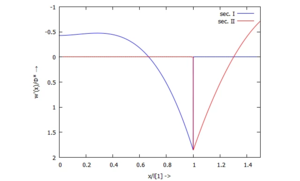
<!--------------------------------------------------------------------------------> | Zum Auftragen der Ergebnisse nutzen wir die Standard-Lösungen - Lastfall 3 mit der maximalen Auslenkung | ||
<math>W^* = \displaystyle \frac{5\;\ell_1^4\cdot q_0}{384 \; EI_1}</math> | |||
und dem Winkel | |||
<math>\displaystyle \Phi^* = \frac{q_0\;\ell_1^3}{24\;EI_1}</math> | |||
Die Ergebnisse sind dann ...<!--------------------------------------------------------------------------------> | |||
{{MyCodeBlock|title=Post-Processing | {{MyCodeBlock|title=Post-Processing | ||
|text=Text | |text=Text | ||
Version vom 7. April 2021, 14:42 Uhr
Aufgabenstellung
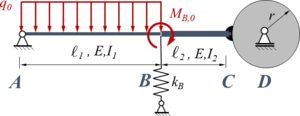
Ein Stab ABC (E-Modul: E) besteht aus zwei Sektionen mit den Längen ℓ1 bzw. ℓ2 sowie den Flächenmomenten I1 bzw. I2. Die Sektionen haben jeweils einen quadratischen Querschnitt, Sektion AB ist durch eine konstante Streckenlast q0 belastet, in B wirkt das Moment MB0. Der Stab ist in A durch ein gelenkiges Festlager gelagert. In C ist das Stabende fest mit dem Umfang einer Rolle vom Radius r verbunden, die in D frei drehbar gelagert ist. In B sind die beiden Sektionen fest miteinander verbunden. Die Feder in B ist eine Translationsfeder mit der Steifigkeit kB.
Interessant ist die kinematische Randbedingung aus der Rolle.
Gesucht ist die Analytische Lösung für den Euler-Bernoulli-Balken und die Verläufe der Schnittgrößen.
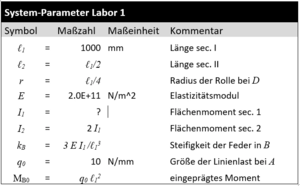
Ermitteln Sie für ein Euler-Bernoulli-Modell die analytischen Verläufe der Schnittgrößen und Verschiebungen im Balken für diese Parameter:
Erstellen Sie dazu ein Programm, mit einem Euler-Bernoulli-Modell für die Berechnung der analytischen Verläufe der Schnittgrößen und Verschiebungen im Balken. Bestimmen Sie Querschnitts-Abmessungen der Sektionen so, dass die maximale Auslenkung des Systems 10 mm beträgt.
Lösung mit Maxima
Die Aufgabe ist ein klassisches Randwertproblem:
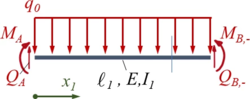
- zwei Gebiete, in denen ein Euler-Bernoulli-Balken in AB und BC durch eine Streckenlast q belastet ist (in Bereich II ist die Streckenlast allerdings Null) und somit durch die Differentialbeziehung
berschrieben wird.
- Rand- und Übergangsbedingungen in den Punkten A, B, C
Wir verwenden xi und ξi als Koordinaten je Bereich, in der Übersicht sieht das Randwertproblem so aus:
| Rand A | Bereich I | Übergang B | Bereich II | Rand C |
|---|---|---|---|---|
 | 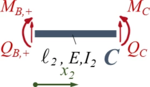 | |||
 |  |  |
tmp
Wir lösen die Feld-Differentialgleichung exakt und passen die Integrationskonstanten an die Rand- und Übergangsbedingungen an.
Header
Text
tmp
Wir definieren zunächst die bekannten Parameter zu
.
Weitere brauchen nicht.
Declarations
Text
tmp
In Bereich I und II gilt dieselbe Bewegungs-Differentialgleichung
,
die wir durch Integration lösen und dann bereichsweise anpassen.
So gilt für Bereich II: q0 = 0.
Die allgemeine Lösung ist mit
... für Bereich I:
... für Bereich II:
.
Generic Solutions for Euler-Bernoulli-Beam
Text
tmp
Für die 2*4 = 8 Integrationskonstanten
suchen wir jetzt die passenden Gleichungen aus Rand- und Übergangsbedingungen.
Zur besseren Übersicht nennen wir die Schnitt-Momente und -Kräfte nach den jeweiligen Knotenpunkten A, B, C und fügen als Index ein + / - hinzu, um die Seite (+: rechts vom Knoten, -: links vom Knoten) zu kennzeichnen, wenn diese unterschiedlich sind.
Aus Rand "A"

| Geometrische Randbedingungen
Kraft- und Momenten-Randbedingungen |
Aus Übergang "B"
| Geometrische Randbedingungen
Kraft- und Momenten-Randbedingungen |
Aus Rand "C"
 | Geometrische Randbedingungen
Kraft- und Momenten-Randbedingungen |
Einsetzen liefert 8 Gleichungen
für die Integrationskonstanten.
Boundary Conditions
Text
tmp
Das Gleichungssystem wollen wir als
schreiben, also
.
Die Matrix-Elemente sind für die Koeffizientenmatrix
und für die rechte Seite
Prepare for Solver
Text
tmp
Das Lösen des Gleichungssystems liefert
Solving
Text
tmp
Zum Auftragen der Ergebnisse nutzen wir die Standard-Lösungen - Lastfall 3 mit der maximalen Auslenkung
und dem Winkel
Die Ergebnisse sind dann ...
Post-Processing
Text
Links
- ...
Literature
- ...