Gelöste Aufgaben/Kit5: Unterschied zwischen den Versionen
| (Eine dazwischenliegende Version desselben Benutzers wird nicht angezeigt) | |||
| Zeile 15: | Zeile 15: | ||
In ''B'' hält das verschiebliche Lager den Balken horizontal. Gegen über Punkt A hält das Lager in B den Balken in einem vertikalen Abstand von W zur Horizontalen. | In ''B'' hält das verschiebliche Lager den Balken horizontal. Gegen über Punkt A hält das Lager in B den Balken in einem vertikalen Abstand von W zur Horizontalen. | ||
<onlyinclude>[[ | <onlyinclude>[[Datei:Kit5.png|250px|left|mini|Lageplan|verweis=Special:FilePath/Datei:Kit5.png.png]] | ||
Gesucht ist die Biegelinie des Balkens, der durch geometrische Randbedingungen vorverformt ist. | Gesucht ist die Biegelinie des Balkens, der durch geometrische Randbedingungen vorverformt ist. | ||
</onlyinclude> | </onlyinclude> | ||
| Zeile 22: | Zeile 22: | ||
In [[Gelöste Aufgaben/Kit4|Kit4]] finden Sie die Transformation der Bewegungsgleichung des Euler-Bernoulli-Balkens in die dimensionslose Form mit der allgemeinen Lösung | In [[Gelöste Aufgaben/Kit4|Kit4]] finden Sie die Transformation der Bewegungsgleichung des Euler-Bernoulli-Balkens in die dimensionslose Form mit der allgemeinen Lösung | ||
<math>\displaystyle {\tilde{w}_{i}}\left( \xi\right) :=\frac{\mu\cdot {{\xi}^{4}}}{24}+\frac{{{C}_{i,3}}\cdot {{\xi}^{3}}}{6}+\frac{{{C}_{i,2}}\cdot {{\xi}^{2}}}{2}+{{C}_{i,1}}\cdot \xi+{{C}_{i,0}}</math> | ::<math>\displaystyle {\tilde{w}_{i}}\left( \xi\right) :=\frac{\mu\cdot {{\xi}^{4}}}{24}+\frac{{{C}_{i,3}}\cdot {{\xi}^{3}}}{6}+\frac{{{C}_{i,2}}\cdot {{\xi}^{2}}}{2}+{{C}_{i,1}}\cdot \xi+{{C}_{i,0}}</math> | ||
für die Bereiche i=1 (''A-B'') und i=2 (''B-C''). | für die Bereiche i=1 (''A-B'') und i=2 (''B-C''). | ||
| Zeile 30: | Zeile 30: | ||
Die Rand- und Übergangsbedingungen lauten dann mit ''ℓ<sub>2</sub> = ℓ<sub>1</sub>/2'' | Die Rand- und Übergangsbedingungen lauten dann mit ''ℓ<sub>2</sub> = ℓ<sub>1</sub>/2'' | ||
<math>\begin{array}{ccl} \tilde{w}_1(0)&=&0\\\tilde{w}'_1(0)&=&1/10\end{array}</math>, <math>\begin{array}{ccl} \tilde{w}_1(1)&=&\tilde{w}_2(0)\\\tilde{w}'_1(1)&=&\tilde{w}'_2(0)\\\tilde{w}''_1(1)&=&\tilde{w}''_2(0)\\\tilde{w}'''_1(1)&=&\tilde{w}'''_2(0)\end{array}</math>, <math>\begin{array}{ccl} \tilde{w}_1(1/2)&=&W\\ \tilde{w}'_2(1/2)&=&0\end{array}</math> | ::<math>\begin{array}{ccl} \tilde{w}_1(0)&=&0\\\tilde{w}'_1(0)&=&1/10\end{array}</math>, <math>\begin{array}{ccl} \tilde{w}_1(1)&=&\tilde{w}_2(0)\\\tilde{w}'_1(1)&=&\tilde{w}'_2(0)\\\tilde{w}''_1(1)&=&\tilde{w}''_2(0)\\\tilde{w}'''_1(1)&=&\tilde{w}'''_2(0)\end{array}</math>, <math>\begin{array}{ccl} \tilde{w}_1(1/2)&=&W\\ \tilde{w}'_2(1/2)&=&0\end{array}</math> | ||
= | <!--------------------------------------------------------------------------------> | ||
{{MyCodeBlock|title=Lösen der dimensionslosen Bewegungsgleichung | |||
|text= | |||
Damit es einfacher wird, lassen Tilde über ''w'' weg. Die Bewegungsgleichung für beide Bereiche ist dann | Damit es einfacher wird, lassen Tilde über ''w'' weg. Die Bewegungsgleichung für beide Bereiche ist dann | ||
<math>w''''(\xi)=\mu</math> | ::<math>w''''(\xi)=\mu</math> | ||
und deren allgemeine Lösung | und deren allgemeine Lösung | ||
<math>\begin{array}{l} \displaystyle {{w}_{1}}\left( \xi\right) :=\frac{\mu\cdot {{\xi}^{4}}}{24}+\frac{{{C}_{1,3}}\cdot {{\xi}^{3}}}{6}+\frac{{{C}_{1,2}}\cdot {{\xi}^{2}}}{2}+{{C}_{1,1}}\cdot \xi+{{C}_{1,0}} \\ \displaystyle {{w}_{2}}\left( \xi\right) :=\frac{{{C}_{2,3}}\cdot {{\xi}^{3}}}{6}+\frac{{{C}_{2,2}}\cdot {{\xi}^{2}}}{2}+{{C}_{2,1}}\cdot \xi+{{C}_{2,0}} \text{ (hier ist } \mu=0 )\end{array}</math> | ::<math>\begin{array}{l} \displaystyle {{w}_{1}}\left( \xi\right) :=\frac{\mu\cdot {{\xi}^{4}}}{24}+\frac{{{C}_{1,3}}\cdot {{\xi}^{3}}}{6}+\frac{{{C}_{1,2}}\cdot {{\xi}^{2}}}{2}+{{C}_{1,1}}\cdot \xi+{{C}_{1,0}} \\ \displaystyle {{w}_{2}}\left( \xi\right) :=\frac{{{C}_{2,3}}\cdot {{\xi}^{3}}}{6}+\frac{{{C}_{2,2}}\cdot {{\xi}^{2}}}{2}+{{C}_{2,1}}\cdot \xi+{{C}_{2,0}} \text{ (hier ist } \mu=0 )\end{array}</math> | ||
|code= | |code= | ||
<syntaxhighlight lang="lisp" line start=1> | <syntaxhighlight lang="lisp" line start=1> | ||
1 | /* solve dim'less dgl (see "Dimensionen und Einheiten") ....*/ | ||
dgl : diff(w(xi),xi,4) = mu; | |||
/* generic solution */ | |||
displ : expand(solve(integrate( | |||
integrate( | |||
integrate( | |||
integrate(dgl,xi),xi), | |||
xi), | |||
xi), w(xi))); | |||
/* adapt to section 1 (AB) und section 2 (BC) */ | |||
sections: [[i=1, | |||
%c4=C[1,0], %c3=C[1,1], %c2=C[1,2], %c1=C[1,3]], | |||
[i=2, | |||
%c4=C[2,0], %c3=C[2,1], %c2=C[2,2], %c1=C[2,3], | |||
mu = 0]]; | |||
/* section I */ | |||
define( w[1](xi), subst(sections[1],subst(displ,w(xi)))); | |||
/* section II */ | |||
define( w[2](xi), subst(sections[2],subst(displ,w(xi)))); | |||
</syntaxhighlight> | </syntaxhighlight> | ||
}} | }} | ||
= | <!--------------------------------------------------------------------------------> | ||
{{MyCodeBlock|title=Formulation of Boundary Conditions | |||
|text= | |||
Einsetzten der Lösung der Bewegungsgleichungen in die Randbedingungen liefert die Gleichungen | Einsetzten der Lösung der Bewegungsgleichungen in die Randbedingungen liefert die Gleichungen | ||
<math>\begin{pmatrix}{{C}_{1,0}}=0\\ {{C}_{1,1}}=\displaystyle \frac{1}{10}\\ \displaystyle \frac{\mu}{24}+\frac{{{C}_{1,3}}}{6}+\frac{{{C}_{1,2}}}{2}+{{C}_{1,1}}+{{C}_{1,0}}={{C}_{2,0}}\\ \displaystyle \frac{\mu}{6}+\frac{{{C}_{1,3}}}{2}+{{C}_{1,2}}+{{C}_{1,1}}={{C}_{2,1}}\\ | ::<math>\begin{pmatrix}{{C}_{1,0}}=0\\ {{C}_{1,1}}=\displaystyle \frac{1}{10}\\ \displaystyle \frac{\mu}{24}+\frac{{{C}_{1,3}}}{6}+\frac{{{C}_{1,2}}}{2}+{{C}_{1,1}}+{{C}_{1,0}}={{C}_{2,0}}\\ \displaystyle \frac{\mu}{6}+\frac{{{C}_{1,3}}}{2}+{{C}_{1,2}}+{{C}_{1,1}}={{C}_{2,1}}\\ | ||
\displaystyle \frac{\mu}{2}+{{C}_{1,3}}+{{C}_{1,2}}={{C}_{2,2}}\\ \mu+{{C}_{1,3}}={{C}_{2,3}}\\ \displaystyle \frac{{{C}_{2,3}}}{8}+\frac{{{C}_{2,2}}}{2}+{{C}_{2,1}}=0\\ \displaystyle \frac{{{C}_{2,3}}}{48}+\frac{{{C}_{2,2}}}{8}+\frac{{{C}_{2,1}}}{2}+{{C}_{2,0}}=1\end{pmatrix}</math> | \displaystyle \frac{\mu}{2}+{{C}_{1,3}}+{{C}_{1,2}}={{C}_{2,2}}\\ \mu+{{C}_{1,3}}={{C}_{2,3}}\\ \displaystyle \frac{{{C}_{2,3}}}{8}+\frac{{{C}_{2,2}}}{2}+{{C}_{2,1}}=0\\ \displaystyle \frac{{{C}_{2,3}}}{48}+\frac{{{C}_{2,2}}}{8}+\frac{{{C}_{2,1}}}{2}+{{C}_{2,0}}=1\end{pmatrix}</math> | ||
mit den Unbekannten | mit den Unbekannten | ||
<math>\underline{x} = \begin{pmatrix}{{C}_{1,0}}\\ {{C}_{1,1}}\\ {{C}_{1,2}}\\ {{C}_{1,3}}\\ {{C}_{2,0}}\\ {{C}_{2,1}}\\ {{C}_{2,2}}\\{{C}_{2,3}}\end{pmatrix}</math>. | ::<math>\underline{x} = \begin{pmatrix}{{C}_{1,0}}\\ {{C}_{1,1}}\\ {{C}_{1,2}}\\ {{C}_{1,3}}\\ {{C}_{2,0}}\\ {{C}_{2,1}}\\ {{C}_{2,2}}\\{{C}_{2,3}}\end{pmatrix}</math>. | ||
|code= | |code= | ||
<syntaxhighlight lang="lisp" line start=1> | <syntaxhighlight lang="lisp" line start=1> | ||
1 | /* formulation of boundary conditions */ | ||
bc : flatten( | |||
[w[1](0) = 0, | |||
subst([xi=0],diff(w[1](xi),xi))=1/10, | |||
makelist(subst([xi=1],diff(w[1](xi),xi,j)) | |||
=subst([xi=0],diff(w[2](xi),xi,j)),j,0,3), | |||
subst([xi=1/2],diff(w[2](xi),xi))=0, | |||
w[2](1/2) = 1]); | |||
/* and unknowns*/ | |||
ic : flatten(makelist(makelist(C[i,j],j,0,3),i,1,2)); | |||
</syntaxhighlight> | </syntaxhighlight> | ||
}} | }} | ||
= | <!--------------------------------------------------------------------------------> | ||
{{MyCodeBlock|title=The Equations of Motion | |||
|text= | |||
Das Gleichungssystem | Das Gleichungssystem | ||
<math>\underline{\underline{A}}\cdot\underline{x} = \underline{b}</math> | ::<math>\underline{\underline{A}}\cdot\underline{x} = \underline{b}</math> | ||
hat dabei die Koeffizientenmatrix | hat dabei die Koeffizientenmatrix | ||
<math>\underline{\underline{A}} = \begin{pmatrix} 1 & 0 & 0 & 0 & 0 & 0 & 0 & 0\\ 0 & 1 & 0 & 0 & 0 & 0 & 0 & 0\\ 1 & 1 & \frac{1}{2} & \frac{1}{6} & -1 & 0 & 0 & 0\\ 0 & 1 & 1 & \frac{1}{2} & 0 & -1 & 0 & 0\\ 0 & 0 & 1 & 1 & 0 & 0 & -1 & 0\\ 0 & 0 & 0 & 1 & 0 & 0 & 0 & -1\\ 0 & 0 & 0 & 0 & 0 & 1 & \frac{1}{2} & \frac{1}{8}\\ 0 & 0 & 0 & 0 & 1 & \frac{1}{2} & \frac{1}{8} & \frac{1}{48}\end{pmatrix} | ::<math>\underline{\underline{A}} = \begin{pmatrix} 1 & 0 & 0 & 0 & 0 & 0 & 0 & 0\\ 0 & 1 & 0 & 0 & 0 & 0 & 0 & 0\\ 1 & 1 & \frac{1}{2} & \frac{1}{6} & -1 & 0 & 0 & 0\\ 0 & 1 & 1 & \frac{1}{2} & 0 & -1 & 0 & 0\\ 0 & 0 & 1 & 1 & 0 & 0 & -1 & 0\\ 0 & 0 & 0 & 1 & 0 & 0 & 0 & -1\\ 0 & 0 & 0 & 0 & 0 & 1 & \frac{1}{2} & \frac{1}{8}\\ 0 & 0 & 0 & 0 & 1 & \frac{1}{2} & \frac{1}{8} & \frac{1}{48}\end{pmatrix} | ||
</math> | </math> | ||
sowie die rechte Seite | sowie die rechte Seite | ||
<math>\underline{b} = \begin{pmatrix}0\\ \frac{1}{10}\\ -\frac{\mu}{24}\\ -\frac{\mu}{6}\\ -\frac{\mu}{2}\\ -\mu\\ 0\\ 1\end{pmatrix}</math> | ::<math>\underline{b} = \begin{pmatrix}0\\ \frac{1}{10}\\ -\frac{\mu}{24}\\ -\frac{\mu}{6}\\ -\frac{\mu}{2}\\ -\mu\\ 0\\ 1\end{pmatrix}</math> | ||
|code= | |code= | ||
<syntaxhighlight lang="lisp" line start=1> | <syntaxhighlight lang="lisp" line start=1> | ||
/* Linear Equations of Motion */ | |||
ACM : augcoefmatrix(bc,ic); | |||
A : submatrix(ACM,9); | |||
b : -col(ACM,9); | |||
</syntaxhighlight> | </syntaxhighlight> | ||
}} | }} | ||
= | |||
<!--------------------------------------------------------------------------------> | |||
{{MyCodeBlock|title=Solving | |||
|text= | |||
Wir erhalten | Wir erhalten | ||
<math>[{{C}_{1,0}}=0,{{C}_{1,1}}=\frac{1}{10},{{C}_{1,2}}=\frac{72+5\cdot \mu}{30},{{C}_{1,3}}=-\frac{444+95\cdot \mu}{135},{{C}_{2,0}}=\frac{2436+25\cdot \mu}{3240},{{C}_{2,1}}=-\frac{5\cdot \mu-231}{270},{{C}_{2,2}}=-\frac{24+\mu}{27},{{C}_{2,3}}=\frac{40\cdot \mu-444}{135}]</math>. | ::<math>[{{C}_{1,0}}=0,{{C}_{1,1}}=\frac{1}{10},{{C}_{1,2}}=\frac{72+5\cdot \mu}{30},{{C}_{1,3}}=-\frac{444+95\cdot \mu}{135},{{C}_{2,0}}=\frac{2436+25\cdot \mu}{3240},{{C}_{2,1}}=-\frac{5\cdot \mu-231}{270},{{C}_{2,2}}=-\frac{24+\mu}{27},{{C}_{2,3}}=\frac{40\cdot \mu-444}{135}]</math>. | ||
|code= | |code= | ||
<syntaxhighlight lang="lisp" line start=1> | <syntaxhighlight lang="lisp" line start=1> | ||
1 | /* solve .... */ | ||
sol[1] : solve(bc,ic); | |||
</syntaxhighlight> | </syntaxhighlight> | ||
}} | }} | ||
= | <!--------------------------------------------------------------------------------> | ||
{{MyCodeBlock|title=Post-Processing | |||
|text= | |||
[[Datei:Kit5-12.png|mini|w(x)]] | [[Datei:Kit5-12.png|mini|w(x)]] | ||
Die Lösung plotten wir, z.B. für die Streckenlast ''μ=100'': | Die Lösung plotten wir, z.B. für die Streckenlast ''μ=100'': | ||
Die dimensionslose Auslenkung ist jetzt etwas größer als "1" - Sie müssen sich also jetzt überlegen, ob Ihre Theorie noch gültig ist! | Die dimensionslose Auslenkung ist jetzt etwas größer als "1" - Sie müssen sich also jetzt überlegen, ob Ihre Theorie noch gültig ist! | ||
|code= | |code= | ||
<syntaxhighlight lang="lisp" line start=1> | <syntaxhighlight lang="lisp" line start=1> | ||
1+1 | /* for plotting, use mu=100 */ | ||
params: [mu=100]; | |||
sol[2] : expand( | |||
subst(params,subst(sol[1],[w[1](xi),w[2](xi)]))); | |||
/* coordinate-transformation put the functions back "in place"*/ | |||
plot2d ([[parametric, t, subst(t,xi,sol[2][1]), [t,0,1]], | |||
[parametric, 1+t, subst(t,xi,sol[2][2]), [t,0,1/2]]], | |||
[gnuplot_preamble, "set yrange [] reverse"], | |||
[legend, "sec. I", "sec. II"], | |||
[xlabel, "x/l[1]->"], [ylabel, "<-w/W"]); | |||
</syntaxhighlight> | </syntaxhighlight> | ||
}} | }} | ||
<hr/> | <hr/> | ||
Aktuelle Version vom 26. März 2021, 07:09 Uhr
Aufgabenstellung
Bei diesem Randwertproblem wird ein Euler-Bernoulli-Balken (Elastizitätsmodul E, Flächenmoment 2-ten Grades I) mit einer Streckenlast q0 im Bereich A-B belastet. In A ist der Balken fest eingespannt, wobei durch eine Einbau-Ungenauigkeit der Rand im Verhältnis 1:10 geneigt ist.
In B hält das verschiebliche Lager den Balken horizontal. Gegen über Punkt A hält das Lager in B den Balken in einem vertikalen Abstand von W zur Horizontalen.
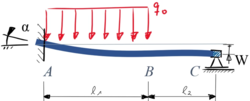
Gesucht ist die Biegelinie des Balkens, der durch geometrische Randbedingungen vorverformt ist.
Lösung mit Maxima
In Kit4 finden Sie die Transformation der Bewegungsgleichung des Euler-Bernoulli-Balkens in die dimensionslose Form mit der allgemeinen Lösung
für die Bereiche i=1 (A-B) und i=2 (B-C).
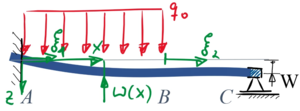
Dazu gehören die neuen dimensionslose Koordinaten ξ1 und ξ2:
Die Rand- und Übergangsbedingungen lauten dann mit ℓ2 = ℓ1/2
- , ,
Lösen der dimensionslosen Bewegungsgleichung
Damit es einfacher wird, lassen Tilde über w weg. Die Bewegungsgleichung für beide Bereiche ist dann
und deren allgemeine Lösung
Formulation of Boundary Conditions
Einsetzten der Lösung der Bewegungsgleichungen in die Randbedingungen liefert die Gleichungen
mit den Unbekannten
- .
The Equations of Motion
Das Gleichungssystem
hat dabei die Koeffizientenmatrix
sowie die rechte Seite
Solving
Wir erhalten
- .
Post-Processing

Die Lösung plotten wir, z.B. für die Streckenlast μ=100:
Die dimensionslose Auslenkung ist jetzt etwas größer als "1" - Sie müssen sich also jetzt überlegen, ob Ihre Theorie noch gültig ist!
Links
- ...
Literature
- ...